The last post argues, not from me but from thirty years’ worth of epidemiological studies, that where we are positioned relative to others matters to our health and happiness.  It matters because we will have more control over our lives and social benefits.

Recall that the title comes from the idea that although absolute status matters, say how much income and education we have, for health and happiness, we have forgotten that the status of others affects us too (relative status).  I will assess the statistics here.

Straightforward Statistics

I view statistics as a way to manipulate the concerns of others, so I will try to pick apart the flagship study from epidemiologists.  We can see that the data is presented as relative risk and not by an odds ratio, which is more intuitive.  However, relative risk exaggerates the significance compared to absolute risk.  But there is not much room to massage things and no statistical inference.  The question then becomes is income, education, or income and education causing the risk of death to change.  The authors conclude that the cause is what income and education bring, which is to increase control and social benefits in our lives.

Explaining the Bar Graph

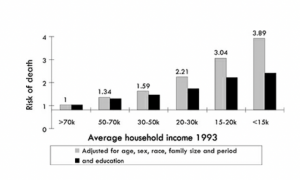
Figure 1: Adapted from data in McDonough (1997)

The above plot is taken from the book “The Status Syndrome” by Michael Marmot.  It is data collected from 1972 to 1991 of a sample size of 8,500 men and women and adjusted to 1993 dollars.  Focusing on the grey bars, the household income of greater than $70k (2021 ~ $140k) was assigned the arbitrary relative risk of death of 1 while, for comparison, the income between $15-$20k (2021 ~ $30-$40k) had a risk of death of 3 times that of the $70k group.  The data are adjusted for age, sex, race, period, and family size while the black bars are adjusted for education.  When we adjust, this means that those things can’t affect the risk of death.  The only thing that is left is education and income—that is, the more status we have, the less risk of death.

For Experts in Statistics

I have real-world experience with uncertainty and accuracy measurements but not with descriptive and inferential statistics from the social and behavioral sciences.  So if anyone does, it would be helpful to understand how the corrections are done to take into account age, sex, race, and education.  I do realize that these are confounding variables or covariants that affect the measurement.  If we want to focus on the relationship of fewer variables affecting the measurement, then we must find a way to correct the data.

References

[1]  Marmot, Michael. The Status Syndrome. Henry Holt and Co.