Last month I heard a lot of buzz about the James Webb Space Telescope. So I made origami of it.
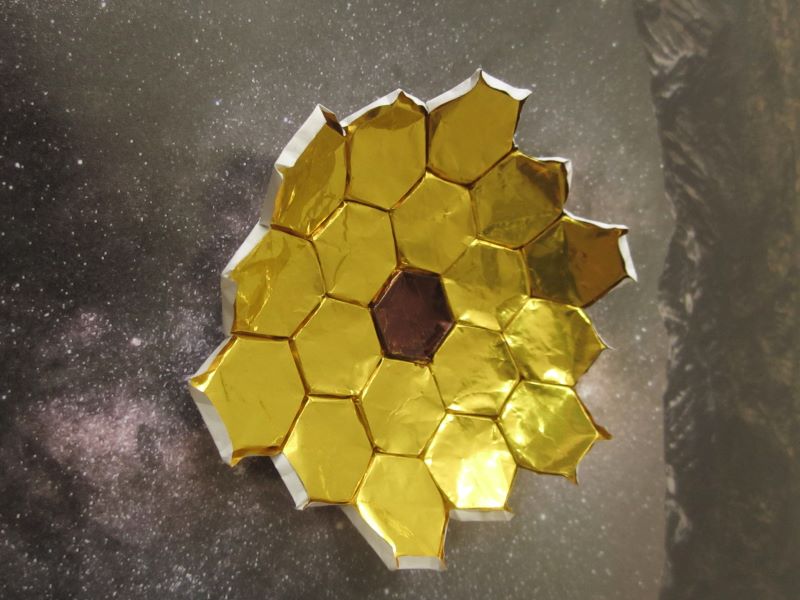
James Webb Telescope, designed by Robert J. Lang. Folding template online.
Specifically, this is just the big mirror component of the James Webb Space Telescope (JWST).
Since people are currently interested in the JWST, and since I just made origami of it, and since I have a physics background, I thought I’d talk about it. Or at least, explain why the mirrors look that way.






