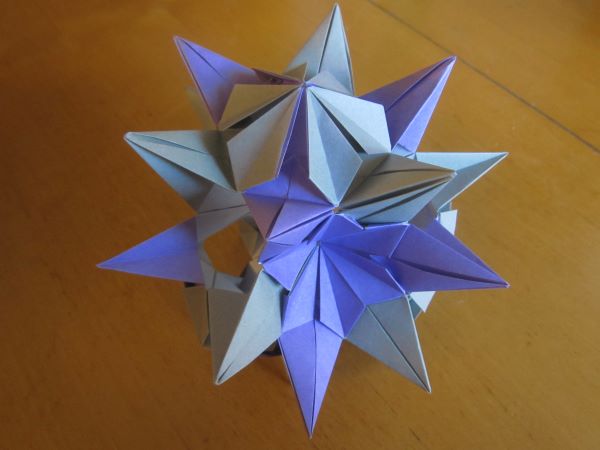
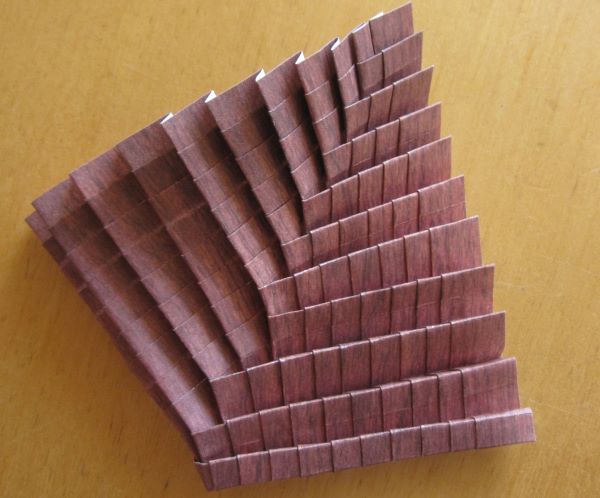
Aperiodic Chevron Tessellation, designed by me
Did you hear? Someone discovered an aperiodic monotile! Obviously, these are origami life goals. And, I’m making it out like a joke, but I’m pretty sure I’m not the only origamist who was thinking that.
Oh, but this origami isn’t the aperiodic monotile. Instead, I read their paper, and was inspired to create a different aperiodic tiling. And in the mean time, I learned how an aperiodic tile ticks.
Bacteriophage, designed (and taught) by Miguel Romero
Last month I went to the local origami convention, and this is one of the models that I learned. Later I folded a second one.
This origami uses a technique called box pleating. Box pleating typically starts by folding the paper into an NxN grid of squares. You then collapse into a base that has the right number of “points”. In this case, there are 6 points for the 6 legs, 2 points are internally hidden, and the head is the 9th point.
This model is simple as far as box pleating goes, but it’s still quite difficult to teach in a convention setting! When you’re familiar with box pleating, you don’t necessarily have explicit steps in your head, you just do it. But most people in the workshop won’t have experience with box pleating, and so the teacher needs to come up with step by step instructions. Even people who are familiar with box pleating may not fold very quickly. So when I say this model is simple, that’s to its benefit. Simplicity is a virtue in origami, especially origami that you teach to others.
Purple Graphite, Based on the “growing polyhedra” schemata in Tomoko Fuse’s Unit Origami
Today’s model is an example of what Tomoko Fuse called “growing polyhedra”. Fuse basically provides a construction kit–designs for triangular double-pyramids and connectors. These components can be put together in any number of ways. I decided to create three layers of hexagons following the crystal structure of graphite–specifically the “ABC” allotrope. This model is quite large, about 8 inches across–and very sturdy too.
Ace and Arrow designed by me, based on “Valentine” by Robert Lang
It’s February, and in the US, the culturally dominant holiday for February is Valentine’s Day. A lot of people don’t like it though, for various reasons that I am sympathetic to. One February, I decided to take Robert Lang’s “Valentine” design, which is a heart with an arrow, and turn it into a spade. I’m pretty happy with this design. I made several of them.
Sparaxis/Bitterroot, designed by Ekaterina Lukasheva
Some context on my origami blogging: I have hundreds of origami photos spanning over a decade. Every month I select one to blog about based on however I happen to be feeling at that moment. You can in fact find all my photos on my Flickr, if you are clever enough to find the link, but that’s a rather different experience.
I don’t follow any pattern in selecting photos, except that I separate the photos that I’ve blogged about and those that I haven’t. One of the consequences of this method is that sometimes it feels like the oldest remaining photos are the dregs. I don’t mean that they’re dregs in the general sense, I mean they’re my personal dregs, the models that I feel least enthusiastic about for one reason or another. So let’s talk about this model from 2014, which I have somehow never selected until now.
Pleated leaf, designed by me, but likely a common design
At some point, I discovered the area of pleat tessellations, a technique popularized by origamist Goran Konjevod. There are a bunch of things you can do with this technique, but I thought I’d show the simplest example I have. This is something that you can fold at home, and experiment with your own variations.
To make this model, we start by folding the paper into 16 divisions both horizontally and vertically. This is a common first step in many origami tessellations, so forgive me for just throwing it out there like it’s nothing. For once, I’ll go into more depth about how to do that.
Rhinoceros, designed by Nguyen Hung Cuong
When I folded this model back in 2016, I was challenging myself to fold it without explicit instructions.
Traditionally, people fold origami by following folding diagrams, which list out the steps in sequential order. However, at more advanced levels, you can fold origami with nothing but a crease pattern, also called a CP. A CP shows where all the essential creases will lie, if the paper were fully unfolded. CPs provide information in a much more condensed format, and are far easier to create than folding diagrams, so it tends to be a lot easier to find CPs than full folding diagrams.
Below the fold I have a few tips for origamists interested in folding from CPs.