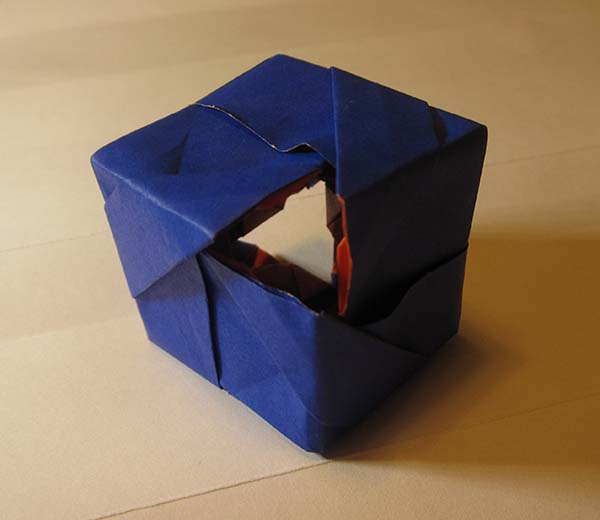
Toroidal Cube, a design by me
In case it’s not clear what’s going on in the photo, the model is in the shape of a cube, and we are looking at it directly onto one of the vertices. However, the vertex has been cut out, and there is a triangular hole in its place. There is also a triangular hole on the opposite end, so that you can see directly through the cube. The triangles aren’t really triangles, but they look triangular from just the right perspective.
Today’s model was inspired by the regular toroid. You may have heard of a torus, which is the shape of a donut, or a mug. A toroidal polyhedron (aka a toroid) is a polyhedron which is also a torus. A regular toroid is a toroidal polyhedron where each face has the same number of sides, and each vertex connects the same number of edges.
The mathematician Laos Szilassi proved that every regular toroid with one hole has faces that are triangles, quadrilaterals, or hexagons. This is easy to understand if you’re familiar with Euler’s formula, which states that V-E+F=2 (where V, E, and F are the number of vertices, edges, and faces). For a torus with one hole, Euler’s formula is modified to V-E+F=0. If each face has A sides, and each vertex connects B edges, then we can show V=F*A/B and E=F*A/2. Thus we have A/B-A/2+1=0. The only possible solutions have A = 3, 4, or 6.
The toroidal cube is in the shape of one of the examples provided by Szilassi. This isn’t the first time I’ve made a regular toroid, I also posted another one last year. Both this model and the other one have A=6.

I made two of these. The one on the right has a slight modification, but I decided I liked the version on the left better.

Uhhh… They really are triangles (messy physical ones, obviously, not ideal geometric objects), in planes that are perpendicular to the direction the camera’s pointing (roughly speaking).
They may not “look triangular” in some other plane, but that’s true of all triangles (planar or otherwise). Of course they could “look like” points or line segments or what have you, if you picked something other than the plane which contains them (a sphere, for instance) and wanted to know which points in the figure are in that particular space or surface or whatever it may be.
But it’d be pretty weird to say that being a triangle is merely the case “from just the right perspective,” since planar figures like these exist in some definite plane or another no matter which perspective you happen to have with respect to them.
@consciousness razor,
I think you’re missing that the “triangles” in question are not planar. They become triangles when you project them onto a particular plane. But in 3-dimensions, they’re irregular hexagons.
Well, okay… I’m sort of nitpicking here, but they don’t somehow “become” something they weren’t before (whenever you or I do something). I wouldn’t say those “aren’t really triangles,” since they’re just as triangular as any other triangle is. What you basically wanted to do was to shift discussion from the projections (which are immediately obvious to the viewer) to the somewhat less-obvious surface of the origami, the toroidal cube, so you can use Euler’s formula. That’s not where the projections live, and those things (not the hexagons) are still triangles which just happen to be elsewhere….
Look at this way: the top of the table in the photo is probably rectangular. Yes? (Or substitute whatever it is; just making a guess here.) It would be odd to argue that it’s not actually rectangular, because we should all focus on the other object in the picture (and confirm some theorems about it). Maybe that is what we should do — it’s an interesting object, and I’d agree we should do that! But that doesn’t change the table. It’s still a rectangle or a circle or whatever, even if our attention should go to something else.
Oh, roll eyes. Sure.