This is a repost of an article I wrote in 2014. Note that Sean Carroll also wrote about this, and he’s an author of the cited paper.
My newest favorite philosophical dilemma is the Sleeping Beauty problem. The experiment goes as follows:
1. Sleeping Beauty is put to sleep.
2. We flip a coin.
3. If the coin is tails, then we wake Sleeping Beauty on Monday, and let her go.
4. If the coin is heads, then we wake Sleeping Beauty on Monday. Then, we put her to sleep and cause her to lose all memory of waking up. Then we wake her up on Tuesday, and let her go.
5. Now imagine Sleeping Beauty knows this whole setup, and has just been woken up. What probability should she assign to the claim that the coin was tails?
There are two possible answers. “Thirders” believe that Sleeping Beauty should assign a probability of 1/3 to tails. “Halfers” believe that Sleeping Beauty has gained no new relevant information, and therefore should assign a probability of 1/2 to tails. The thirder answer is most popular among philosophers.
This has deep implications for physics.
There is an argument that Everettian Quantum Mechanics (aka Many Worlds Interpretation, henceforth EQM) requires that you be a halfer. Say that you tell Sleeping Beauty that you will wake her up on Monday and Tuesday, with a memory wipe in between. The argument goes that this is exactly analogous to telling her that you will cause her wavefunction to branch, and in one branch she will wake up on Monday and in the other on Tuesday. In both cases, the Sleeping Beauty on Monday and Sleeping Beauty on Tuesday are both real, and neither has access to the other (either because of the memory wipe or because they are in separate branches).
Therefore, the standard sleeping beauty problem (“Two-Branch-Beauty” on left) is equivalent to the quantum sleeping beauty problem (“Three-Branch-Beauty” on right).
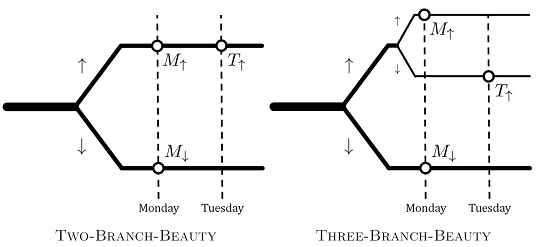
From “Self-Locating Uncertainty and the Origin of Probability in Everettian Quantum Mechanics“. In this paper, they also interpret the very first coin flip as a quantum measurement which causes the wavefunction to branch.
In the Three-Branch-Beauty problem, EQM straightforwardly says that Sleeping Beauty should assign a probability of 1/2 to being in the “tails” branch. If the Three-Branch-Beauty and Two-Branch-Beauty problems are indeed analogous, then she should also assign a probability of 1/2 in the Two-Branch problem. And therefore, if we accept EQM, we must accept the unpopular halfer solution.
The problem with this argument, I think, is that assigning probabilities in EQM is… not at all straightforward. This is actually the major disadvantage of EQM, is that it’s not clear why we should interpret the branching worlds with probabilities. How can we say one branch is more likely than another, if both branches are equally real?
The paper, “Self-Locating Uncertainty and the Origin of Probability in Everettian Quantum Mechanics” purports to answer this question (also see Sean Carroll’s blog about it). When we assign probabilities to branches, these are interpreted as “self-locating” probabilities. That is, even if all branches are real, we still have to ask the question, which branch am I in now? You might naively take the principle of “indifference”, arguing that all branches are equally likely. But that gets you the wrong probabilities.
The paper argues for a different principle, the “Epistemic Separability Principle”, the idea that Sleeping Beauty assigns probabilities on the basis of only what she observes. So if there is a second measurement device that Sleeping Beauty does not look at, then the probability she assigns to the first measurement should be independent of the result of the second measurement. There’s a simple argument on page 4 which shows that if there are two branches of the wavefunction with equal amplitude, then we should assign equal probabilities to each branch.
So let’s say we have the Three-Branch-Beauty experiment, and Sleeping Beauty has just woken up. By the Epistemic Separability Principle, she should assign equal probability to waking up in the first branch, and waking up in the second branch, because those two branches have equal amplitude. The third branch does not have equal amplitude, and based on more mathematical arguments, you can show that the third branch is twice as likely as the other two. Therefore, she would assign probabilities 1/4, 1/4, and 1/2 to the three locations.
Let’s say we have the Two-Branch-Beauty experiment, and Sleeping Beauty has just woken up. By the Epistemic Separability Principle, she should assign equal probability between waking up on Monday in the first branch, and waking up on Monday in the second branch, since those two branches have equal amplitudes. Likewise, she should assign equal probability to waking up on Tuesday in the first branch, and waking up on Monday in the second branch. Therefore, she should assign probabilities of 1/3, 1/3, and 1/3 to the three locations. This recovers the popular thirder answer without giving up EQM.

I think you’ve mixed up heads and tails here. Thirders would say that the probability for the single wake-up case would be 1/3. So tails in Carroll’s case, and heads in yours.
@Rob,
You’re right, I fixed it. Where were you in 2014?
I forget.
I’m sorry, I’ve read your description over several times, and I still can’t wrap my head around the argument that the Thirders are making. The odds of a coin flip are 50-50, and if you don’t have any extra information about the world since then (which Sleeping Beauty doesn’t) then those odds seem obviously unchanged, and all the epistemology in the world won’t alter that.
My gut feeling about the problem is that the whole “Monday/Tuesday + mindwipe” aspect is just window dressing that acts as a distraction and can safely be ignored. If you do that, you end up with a problem like this one:
A researcher leaves the room with a box and a couple of marbles. She comes back in and says that she flipped a coin. If the flip was heads, she put a red marble in the box. If the coin was tails, then she flipped the coin again, and if the second flip was red she put in a red marble, and if it was tails she put in a blue marble.
Given that problem, any third grader could tell you that the odds of what is in the box are 75% red and 25% blue, and also that the odds of the initial coin flip are still 50-50. Can someone explain to me why the Sleeping Beauty problem is different from this one? I just can’t understand why adding fairy tale characters and magical mind wipes and philosophical dithering changes the problem.
This interpretation seems like equivocation and verbal trickery. Let’s call the three waking events TM, HM, and HT for Tails Monday, Heads Monday, and Heads Tuesday. Sure, the odds that TM and HM exist are equal – in fact, they are 50-50. But it’s equivocation to pretend that the odds TM and HM exist are the same as the odds that the waking event she is experiencing is TM or HM. The odds that a waking she experiences is any particular event seem like 50 TM / 25 HM / 25 HT, as you said in the previous paragraph. Any other suggestion seems like flim-flam to me.
No doubt I’m missing some subtleties, but my two cents (probably overvalued):
I’ve never understood the thirder argument.
Suppose SB is asked to simply say “heads” or “tails” each time she wakes. Further suppose she decides to always say “tails” (and remembers that decision, of course). Then the experiment is repeated many times. It’s true that, for a sufficiently large number of repetitions, 2/3 of her utterances will be not correspond to the actual toss outcome. So, going in, if she was told she’d get a dollar for every correct correspondence, and lose a dollar for every wrong one, she should definitely say “heads” every time. N reps would net her $N/2.
However, if she is asked what the probability is that heads came up (which I think is usually how the problem is posed), the correct answer is always 1/2.
If the “heads” protocol was to put to sleep and waken nine times, I guess thirders would become tenthers (?).
Shorter: I don’t think amnesia is a good basis for assigning probabilities, even if is profitable.
———————————————–
I see it as a language problem, not a physics one.
If an electron is prepared in a spin-up state wrt your z axis, and you then measure it along an axis at angle θ to z, the probabilities of measuring spin up and spin down are, respectively, cos²(θ/2) and sin²(θ/2). Those are the coefficients you would assign the relevant branches. Now suppose θ is much less than 1. Then to order θ², the coefficients are
1 – θ²/4 (spin up)
θ²/4 (spin down)
It’s fair to say that before you measure you are far more likely to measure spin up. But applying “more likely” to the final outcome seems dodgy to me. Both are equally real, and the coefficients are simply wave function bookkeeping, telling us which outcome was more likely.
“The argument goes that this is exactly analogous to telling her that you will cause her wavefunction to branch, and in one branch she will wake up on Monday and in the other on Tuesday.”
No comprende, señor. If she is awakened on Monday in one of the branches she is put back to sleep, never to wake up again, i.e., she is killed. That is not part of the original description.
Shouldn’t the equivalent to the three branch model include something like this? If the coin comes up heads, we will flip the coin again. If it comes up tails, we will wake you on Tuesday and let you go. If it comes up heads, we will wake you on Monday, put you back to sleep, and kill you in your sleep.
The thirder argument is Bayesian, based upon what Beauty knows. When she is awakened, she knows that she has been awakened, as well as the original setup. If the coin came up tails, she will be awakened once; if it came up heads, she will be awakened twice. So, given her knowledge that she is awakened, the odds are 2:1 that the coin came up heads.
Equivalently, let her make a bet after each awakening that the coin came up heads, and let her payoff for being right be 1 USD. How much should she bet to make the bets fair? 2 USD. If the coin came up heads she will get 2 USD, 1 USD for each bet, but if it came up tails she will lose 2 USD for the single bet. The bets are fair.
BruceGee @4,
Sleeping Beauty does have additional information. She knows that she does not remember anything since the beginning of the experiment. She knows this would not be the case if the coin were tails and it were Tuesday.
That’s not analogous at all. Imagine instead that we flip a coin (which determines the branch we’re in) followed by a second coin (which determines whether it’s Monday or Tuesday). If either coin was heads, then we put in a red marble (Sleeping Beauty wakes up with no memory). If both coins were heads, then we put in a blue marble (Sleeping Beauty wakes up Tuesday with full memory of Monday). If we see a red marble, what’s the probability that the first coin was tails? It’s 1/3.
(Actually I don’t think a third grader would get that one. It’s one of those probability dilemmas that commonly trips people up, like the Monty Hall problem.)
Bill Spight @6,
I don’t think the eventual fate of Sleeping Beauty in any of the branches is relevant. She is asked about probabilities before she learns her fate.
OK, now I’m really confused. I thought that part of the original problem was that, when she wakes up, she has no way of telling whether it is Heads-Monday, Tails-Monday, or Tails-Tuesday, because before she goes back to sleep on Tails-Tuesday she gets her memory wiped:
If she knows for sure when she wakes up whether it’s Monday or Tuesday, then I don’t even understand the question anymore.
My second sentence above should read: “I thought that part of the original problem was that, when she wakes up, she has no way of telling whether it is Heads-Monday, Tails-Monday, or Tails-Tuesday, because before she goes back to sleep on Tails-Monday she gets her memory wiped”
Brucegee1962 @10,
You got heads and tails backwards. (Just like I did when Rob Grigjanis corrected me.)
Oookay. I read up on this some more on Wikipedia, and I think I get the argument now. You’re right, it’s like my example, with the addendum that you open the box and see that the marble is in fact red. It makes sense now.
And you’re right, it’s a lot like the Monty Hall problem. But it does seem clear now. It’s just that the blue marble is hidden because of the way the problem is phrased. Very clever.
Glad to be of service. FWIW I think my 2014 writing could use some improvement.
Well, if the question is equivalent to “If the experiment is repeated many times, in what fraction of SB’s awakenings will the coin have been tails?”, then I guess I am a thirder. Is that what is meant by asking what probability she should assign? If so, I don’t see the controversy.
It doesn’t seem like you can have it both ways. I mean, you have this nice picture of three branches and all, but at the same time, we’re not supposed to be counting branches and determining what is the frequency of this event or that one. Instead, we’re told that a “rational” person should have their credences set up in a particular way (“should” because this is supposed to be the only rational way to do it), such that the person by some wacky coincidence happens believe both EQM and the Born rule probabilities (so that many worlds isn’t totally dead in the water as a theory), even though those don’t correspond to how common it actually is for this event to happen in the Everettian multiverse, since EQM generally doesn’t appear to offer definite answers about the latter. Your own opening maneuver after setting up the problem was to claim that a straightforward approach of counting branches doesn’t work and so perhaps we ought to look for something else, if we still have any reason to keep bothering with EQM. (That to me seems like a problem for EQM to wrestle with, not a problem with the sleeping beauty argument itself.)
Maybe I simply don’t get it, but it just doesn’t sound like it’s explaining the experimental outcomes it’s apparently attempting to explain. Why do we get any probabilities other than 1, if it’s true that all of the outcomes will certainly happen? It’s a bait-and-switch or goalpost-shifting type of move, which substitutes that explanation for the explanation of something else that you might hope that you can pass off as good enough, not something that recovers the right answer properly and lets you keep EQM.
consciousness razor:
Yeah, in the various versions of (neo-)Everettian quantum mechanics, branches are well-defined except when they’re not.
It is improper to leave Tuesday out of any branch, since it appears in one of them. It is apparently left out of the branch where Beauty is awakened only on Monday, but it is implied by the fact that “only”. In that branch Beauty is not awakened on Tuesday. If you leave the day labels out, because Beauty does not know what day it is when awakened, OK. But then the three branch tree has one OR node (the first) and one AND node (the second). Upon what basis do you treat the AND node as an OR node (coin flip)?
Doing so would be OK in a game tree, where each player plays randomly. The first player flips a (fair) coin to decide which branch to take at the OR node. If the coin comes up heads, the second player flips the coin to decide which branch to take at the AND node. This scenario is consistent with assigning 50:50 odds to each branch. However, in this case Beauty is awakened only once, regardless of the original coin flip. So of course to her the odds of heads vs. tails remain 50:50. The three branch tree is deceptive, because it makes it look like the second branch is either/or instead of both/and. There is no 50:50 choice at the second branch.
Game theory does offer a clue, however. Beauty’s state of knowledge is the same after each awakening. This may be indicated by putting all of them in the same enclosure. That means that Beauty’s strategy is the same after each awakening. But what are Beauty’s choices? To answer the question of whether the coin came up “Heads” or “Tails”. What are her payoffs? To be right or wrong. Let’s evaluate those payoffs in dollar terms. Say that if she is right, she gets 1 USD, if she is wrong, she loses 1 USD. Then her correct strategy is to say that the coin came up Heads. Her expected payoff is 50 cents. Half the time she loses 1 USD, half the time she wins 2 USD. Nobody should take that bet.
Let’s make the game slightly more complicated. Let Beauty offer odds, instead of making a 50: 50 bet. As we have seen, she should offer 2:1 odds to make the bet fair. That implies 2:1 odds that the coin came up Heads. But instead, let Beauty use a mixed strategy with an even odds bet, claiming that the coin came up Heads with a certain probability, P, and Tails with probability, 1-P. If she announces a P > ⅔, she should get no takers, if she announces a P < ⅔, she will be at a disadvantage if her offer is taken. The only fair bet occurs with P = ⅔. Again, that implies a probability of ⅔ that the coin came up heads.
Note that all of these bets assume a fair coin, one with a probability of coming up Heads half the time. There is no inconsistency between that probability and the probability that the coin came up heads each time Beauty is awakened. What the second probability has to do with QM, I cannot say. It may well be that QM is concerned only with the first.
Sorry. I was wrong about the mixed strategy. The proper mixed strategy is 50:50. Regardless of how the coin came up, the expectation is 0. Even if the coin is two headed or two tailed.
Isn’t Tuesday heads dependent on Monday heads, so how can they exist in separate branches?
Seems to me the probability of waking up on a Monday is 2/3, but the probability of tails is 1/2
Write “Monday” on two pieces of paper. Add “Heads” to one of them, and “Tails” to the other. Repeat with two more pieces of paper, but start with “Tuesday.” Put the four pieces of paper in a hat, and have SB pick one. Repeat your experiment, waking SB once (on the day she picked if the coin landed on the side she picked) or twice (on Monday and Tuesday if the coinis didn’t land on the side she picked). What probability should she assign to the claim that the coin landed on the side she picked?
This is your version if she picked “Tuesday, Tails.” The more traditional version is “Tuesday, Heads.” Regardless, the answer is the same no matter what she picks, or even if she isn’t shown what she picked.
Now use four SBs, and have each pick a different piece of paper. On Monday you will wake three of them, leaving out the one who picked “Monday” and the actual coin result. Each can easily see that the chances that her piece of paper says the actual result are 1 in 3. The same is true on Tuesday.
The wording above suggests another way to prove 1/3, and what is wrong with the halfer argument:
The description requires a four-branch system. Three of the branches are the same as in the three-branch quantum mechanics figure above. The fourth is for Tuesday, when Beauty is at a spa spending the modest compensation she received for subjecting herself to this experiment.
The error in the halfer interpretation, is not defining “new information.” Its existence does not depend on how she would know that it is Tuesday, after tails. It depends only on being in a state that she knows isn’t. The transition from Monday to Tuesday still occurs. Beauty has direct evidence that she is in one of three equally-likely branches, because she is not in the fourth. The EQT model is invalid unless the is a second state change in both paths from the first, and the state in that path is distinguishable.
And this is true even if we keep Beauty asleep thru Tuesday. That is still a state. The answer is 1/3