Image source: Wikipedia

The light mill, also known as the Crookes radiometer, is a little curiosity that can be found in stores at science museums, or in the offices of physics professors. It consists of four vanes, each painted white on one side, and black on the other. The vanes are placed in a bulb, under partial vacuum. When you shine light on the radiometer, the vanes spin around, with the white sides facing forward (counter-clockwise in the above image). A demonstration is shown in the video below.

Supposedly, the Crookes radiometer is an educational tool, but I can’t for the life of me determine what lesson it’s supposed to teach. Because if you ask how it works, the correct explanation is far beyond the ability for most people to understand. I have a Ph.D. in physics, and I still couldn’t understand it! There are numerous internet sources that identify “thermal transpiration” as the correct explanation, but fail to explain what that means or why it happens. So instead I found explanations in scientific literature,¹ and now I will share the explanation with you.

Basic explanations, and why they don’t work

When Sir William Crookes invented this radiometer in 1874, his initial explanation was that the spinning was caused by radiation pressure. Whenever you shine light on an object, the light imparts a small force on the object. James Maxwell had predicted radiation pressure in 1862, and Crookes thought he had come across a neat demonstration of it.

However, this mechanism doesn’t work, for two reasons. First of all, radiation pressure is far too weak. Second of all, it’s in the wrong direction. Radiation pressure would apply more force to the white side of the vanes, because of the recoil force of light reflecting off of the white side.²

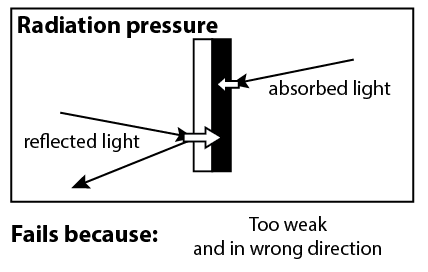
Another explanation that was considered, was the outgassing of particles from the vanes. Because light is absorbed by the dark side, we expect that the dark side will be hotter, and thus outgas particles at a higher rate. The recoil from the outgassed particles would push the dark side.

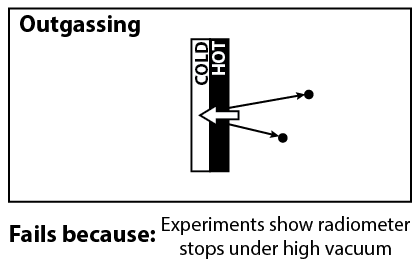
Unfortunately, this can be ruled out with further experiments, demonstrated in the video above. The Crookes radiometer works best under partial vacuum, with the optimal pressure about 10^-5 atm. If outgassing were the correct mechanism, then the radiometer would work even under perfect vacuum.

Failed thermodynamic explanations

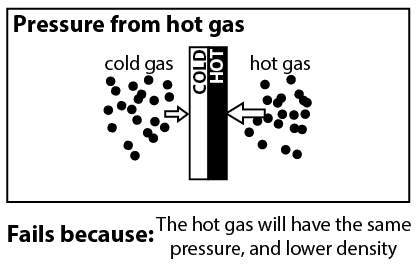
Now that we understand that the radiometer only works when there’s some gas in the bulb, we can look at explanations that rely on the thermodynamics of gasses. When we shine light on the radiometer, the black side of the vanes become hotter than the white sides.³ And you might think, don’t hot gasses have higher pressure than colder gasses? If the air around the black side has higher pressure, then it will push the black side, causing the radiometer to spin.

Unfortunately, it is not true that hot gasses have higher pressure than cold gasses. According to the ideal gas law,⁴

P = nkT

where P is pressure, T is temperature, n is the density of molecules, and k is the Boltzmann constant. If n is unchanged, then a hotter gas will indeed have a higher pressure. However there is no reason to expect n to be unchanged. If it were the case that the hot region of gas had higher pressure, then this pressure would push gas molecules out of the region until it no longer had higher pressure

Another clever idea is to note that gas particles that hit the vanes may bounce back with a different speed than what they started with. On average, gas molecules that hit the black side will recoil with a greater speed, and molecules that hit the white side will recoil with a lower speed. But this mechanism fails for the same reason. If molecules are recoiling with greater speed, then they also have greater ability to push other molecules away, thus decreasing the local density of air molecules.

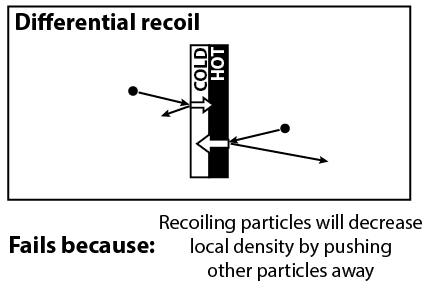
On a side note, I believe that this mechanism might have an effect if the density of gas is so small that molecules practically never collide with each other. We can check this by looking up the mean free path length, which is the average distance that molecules will travel before having a collision. We can say that the molecules practically never collide if the mean free path length is much larger than the size of the bulb. Unfortunately, the size of the bulb is about 10 cm, and the radiometer works best for a mean free path length from 1 mm to 1 cm. (And if you watched the video, there was a demonstration in an even bigger bulb.) The fact that we don’t see an effect at lower pressures suggests that the recoil effect is too small to generate motion.

Thermal Creep and the Einstein effect

Finally, we get to the explanations that work! First, there is thermal transpiration (called thermal creep by modern researchers), proposed by Osborne Reynolds in 1878. Second, there is the Einstein effect, proposed by Einstein in 1924. We’ll start with Einstein, since that one is simpler.

To understand the Einstein effect, let’s start with a thought experiment. Suppose that the radiometer bulb has a diffuse gas on one side of the vane, and is under perfect vacuum on the other side of the vane.  We want to estimate the pressure that the gas applies to the vane, and the flow of the gas around the vane. To perform this estimate, we need to know that the root mean square velocity (v) of the gas particles is proportional to sqrt(T).

The pressure of the gas is a product of two things: how many molecules are hitting the vane per unit area, and how hard they’re hitting. The number of particles per unit area is proportional to nv; how hard the particles are hitting the barrier is proportional to v. Thus we estimate that the pressure is proportional to nv², or nT.  (If we know that the constant of proportionality is k, then we can reproduce the ideal gas law, P = nkT.)

The flow of the gas depends only on how many molecules are crossing the vane per unit area; it does not depend on how hard they’re hitting. Thus, the flow is proportional to nv, or n*sqrt(T).

Now, we consider the more realistic picture, where there is gas on either side of the vane, but the gas on one side is hotter than the gas on the other side. When we look at the gas near the center of the vane, the pressure will equalize, so we’ll have n₁T₁ = n₂T₂. But when we look at the gas away from the vane, it’s not the pressure that equalizes, but the flow. So we’ll have n₁sqrt(T₁) = n₂sqrt(T₂). At the edges of the vane, there must be some zone of transition between the two distinct behaviors (the width of the transition region being the mean free path length). In this transition region, you expect that neither the flow nor the pressure quite equalize. There is a net flow from the white side to the black side, and a net pressure on the black side. This causes the vanes to rotate.

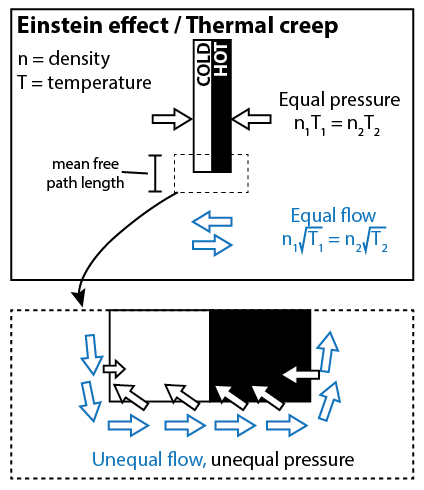
This effect only occurs near the edges of the vanes, with a distance no more than the mean free path length. As I said before, the Crookes radiometer works best when the mean free path length is around 1 mm to 1 cm. Which is around the size of the vane itself, so that makes sense. If you were to poke a bunch of holes in the vane, in theory it would work even better.

The Einstein effect is quite complicated, and you might be bracing yourself for an even more complicated explanation of thermal creep. But this is unnecessary. As far as I can tell, thermal creep and the Einstein effect are the same! At least, this is the claim made by Scandurra,¹ who says the Einstein effect is essentially the same as thermal creep, except that Einstein uses a toy model, whereas thermal creep is based on non-equilibrium statistical equations.  (I also found conflicting accounts, but at the risk of being wrong, I’m siding with Scandurra, who had more compelling arguments.⁵)

Thermal creep describes the gas “creeping” along the surface of the vane, near its edges. When gas molecules hit the surface, on average they get pushed towards the hot side, which contributes to the creeping motion. And in the process, the vane gets pushed backwards, towards the cold side. As for why the gas molecules get pushed towards the hot side… Because math? For a less opaque explanation, I refer back to the Einstein effect.

What did we learn?

Even really simple physics demos can be really tough to explain.

Footnotes

1. Scandurra, M. Enhanced radiometric forces. (2008). https://arxiv.org/abs/physics/0402011v1
(return)

2. John Baez discusses the fact that the black side of the vane is also emitting heat radiation.  So if we assume a perfect vacuum, and perfect insulation between the white and black sides, then the radiation pressure will all cancel out!  However, in a realistic experiment I expect that those assumptions are not perfect, and the forces do not cancel out.  And in any case, the forces are still too small. (return)

3. If you watched the video, you saw that when the bulb is cooled, the radiometer rotates in the opposite direction. This is because even when there’s no external light source, all objects emit and absorb heat radiation. When the bulb is cold, the vanes emit more heat radiation than they absorb, and dark objects tend to emit heat radiation at faster rates. (You can’t see the heat radiation because it’s infrared.)  As a result, the black side will be cooler than the white side, which is the opposite of the normal situation. So whatever mechanism we have for the Crookes radiometer, we expect the same mechanism to act in reverse. (return)

4. Some readers may be familiar with another form of the ideal gas law, with PV = nRT. The equation I show is the same, but dividing out V, and using different units. In “PV = nRT”, n represents the number of moles of gas molecules in the container, while in “P = nkT”, n represents the density of gas molecules. This is standard notation; I’m not to blame for the fact that a single letter represents two things. (return)

5. For example, I found a recent study⁶ which used a Crookes radiometer with horizontal vanes, arguing that this only had the thermal creep effect, and no Einstein effect. This implies a belief that they are distinct effects. However, they did not present any evidence or argument that they are distinct.

The current Wikipedia article also claims that the Einstein effect and thermal creep are distinct and competing explanations. But there is no citation for this, and I managed to trace it to a single anonymous editor from 2004.

Also, Wikipedia’s explanation of the Einstein effect is essentially differential recoil effect as applied to the edges, which seems rather different from my explanation (which comes from Scandurra).  On the other hand, perhaps this is just a different way of describing the same thing?  Consider gas particles that are hitting the edge of the vane at an oblique angle.  If those particles are coming from the hot side, then they will be hotter than the surface they hit, so they bounce off with lower speed.  If they’re coming from the cold side, then they will be colder than the surface they hit, so they bounce off with higher speed.  But this is just guesswork on my part.  (return)

6. Wolfe, D., Larraza, A., and Garcia, A. A horizontal vane radiometer: Experiment, theory, and simulation. Physics of Fluids 28, 037103 (2016). https://doi.org/10.1063/1.4943543 or https://arxiv.org/abs/1512.02590 (return)