This is a repost of an article I wrote in 2013, back when I was willing to take the time to explain physics stuff, and generate lots of images too.
It’s often said that topology is the branch of mathematics where they can’t tell the difference between a donut and a coffee mug. They each have a single hole (the mug’s handle and the donut hole), and that’s all that matters. If I may overanalyze this joke, the point seems to be that topology is so disconnected from our everyday experience. How is this useful?
I wish to explain one particular use of topology in physics: topological defects.
A topological defect is a sort of knot that exists in the microscopic structure of a material.* You can move the knot around from atom to atom, but you can’t untie it. We’ll get into how that works soon enough.
*Material is a vague term for “stuff”. Later I’ll discuss a few different materials including magnets, liquid crystals, and superconductors
2D Magnets
One classical material that everyone is familiar with are permanent magnets (aka ferromagnets). Permanent magnets are made of lots of little atoms, each of which generates a tiny magnetic field. In fact, many materials contain atoms that produce magnetic fields.. The miracle of permanent magnets is that all the magnetic fields of the atoms align together in the same direction.

(All images in this post, unless otherwise credited, are my one. Use only with attribution.)
Of course, none of the atoms knows which direction is the “right” direction. Rather, each atom interacts with its nearby neighbors. It’s energetically favorable for nearby atoms to align with each other. But if its neighboring atoms happen to be in the “wrong” direction (perhaps because of random motion, or impurities), there’s no way for the atom to know.
Suppose that the atoms were instead in a configuration like one of these:

Both of these configurations are “smooth”, meaning that each atom is pointing in nearly the same direction as its neighbors. However, there seems to be a single discontinuity at the center of each configuration. This is a topological defect. Specifically, this is called a point defect, because it exists at one point. It is not possible to remove the point defect without temporarily sacrificing the overall smoothness. Therefore, even though the topological defects are not the lowest-energy states, they are still somewhat stable.
To demonstrate, let’s draw a loop around the point defect. As we cross each arrow with our loop, take note of the direction of that arrow. You’ll note that the arrows change direction very smoothly (as long as we didn’t draw the loop through the point defect). Once you get back to the beginning of the loop, the direction of the arrow should be the same as where you started. However, in the meantime, the direction of the arrow has gone around completely in a circle!

There’s no way to smoothly deform the arrows so they don’t go around in a circle. To steal an analogy from my professor, it’s like I tied a string in a loop around my finger. I can’t very well take the string off, because my finger is in the way (ignoring the possibility of slipping it off the end of my finger).
Generalizing order
To better understand these topological defects, we have to think about the order parameter manifold. The “order parameter”, in this case, is just the direction of each arrow. The “order parameter manifold” is the set of all possible directions that the arrow can point. In this example, the arrow may point in any direction in a 360 degree circle. Therefore, the order parameter manifold is a circle. If we draw a path around the circle, there is no way to continuously deform the path so that it no longer goes around the circle. That’s why there exists a point defect.
In fact, there will be two kinds of point defects. One defect corresponds to going around the circle clockwise. The other corresponds to going around the circle counter-clockwise. (I showed one of each kind in an image above.) As it happens, if these two point defects meet, they cancel each other out. The defects behave a bit like particles and antiparticles, annihilating when they encounter each other.
Now let’s consider the case where the magnetic field of each atom may point in any direction in three dimensions. In this case, the order parameter manifold is not a circle, but a sphere (specifically, the surface of the sphere). That makes a big difference, because if you draw a loop around the sphere, now it is possible to continuously deform the path into a single point. (In mathematical terms, every loop is “homotopic” to a point.) This means that there is no point defect (in 2D).
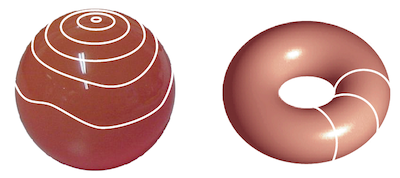
On the other hand, if you had an order parameter manifold which was shaped like a donut, then there would be some loops which you could not shrink down to a point. These loops would correspond to point defects.
Some order parameter manifolds may be even more complicated. For example, liquid crystals are made of long molecules. In the “nematic” phase of liquid crystals, the orientation of the molecules becomes an order parameter. But the order parameter manifold isn’t quite a sphere, because you only need to go around 180 degrees to get back where you started. Instead, it is an exotic topology called the real projective plane. The real projective plane looks like a sphere, except that opposite points of the sphere are identified as the same point. Here is a point defect in a 2D liquid crystal:

Even though I drew all the orientations to be in the plane, they are still free to move out of the plane. But still there is no way to smoothly get rid of the defect.
What’s interesting about defects in a real projective plane is that there are no defects and anti-defects. There is only one kind of defect. If any two of these defects meet, they will annihilate. This makes sense, because one defect corresponds to going around the sphere 180 degrees. Two defects corresponds to going around the sphere 360 degrees.
Other kinds of defects
All the above examples were point defects in 2D materials (though the order parameter manifold may have more dimensions). But there are other kinds of defects in higher-dimensions. For example, there is such a thing as a line defect, also called a vortex (the plural is “vortices”).

(I’m drawing more liquid crystals because my version of Mathematica can’t draw arrows in 3D)
Whenever there would be a point defect in 2D, there will be a vortex in 3D. All it takes is a loop in the order parameter manifold that cannot be continuously deformed to a point.
On the other hand, if there is a bubble in the order parameter manifold that cannot be continuously deformed to a point, then we can have point defects in 3D. One example of such a defect is the “pincushion” pattern.

There’s also another class of defects which aren’t defects at all: topologically nontrivial field configurations. Basically, the order parameter is smooth everywhere, but there’s a funny pattern in the order parameter that cannot be removed. Here is an example in a 2D liquid crystal:
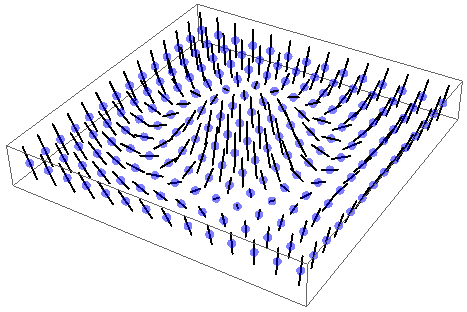
The region in the center can be moved, squeezed, spread out, but it cannot be removed smoothly. Topologically nontrivial field configurations exist in 3D as well, but it would be hard to visualize. Incidentally, if there is a nontrivial field configuration in 2D, then there must be a point defect in 3D. If there is a nontrivial field configuration in 3D, then hypothetically there would be a point defect in 4D.
Superconductors
I admit, I think it’s just cool that topology appears in the real world, even if it’s not so useful. But at the same time, I’m sure it is useful. I study superconductors, which have topological defects of their own. The superconductor’s order parameter manifold is the same as my first example: a circle. (The order parameter, however, is not magnetic field direction, it’s the complex phase of the superconducting condensate’s wavefunction.) So that means that superconductors can have vortices.
And superconducting vortices are really important! As I’ve explained in a previous post, there are type I and type II superconductors. Type I superconductors block any magnetic fields, and a sufficiently strong external magnetic field will destroy the superconductivity. Type II superconductors can typically handle a stronger magnetic field, because they form vortices which the magnetic field can penetrate
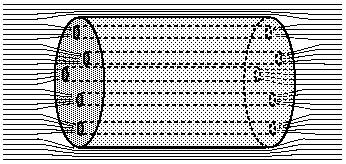
(Image from Hyperphysics)
If we want superconducting wires with high electric current, we need them to tolerate the magnetic fields produced by that current. The superconducting magnets in MRI machines, therefore, require type-II superconductors. Topological defects: saving lives!


Thanks Siggy. Next: the renormalization group? 🙂
No I don’t think I could explain RG theory.